Archery is a sport that is good for the mind and the body, greatly benefiting my health and well-being. But it's also got something for me in other ways. One of the reasons why I choose archery over many of the other Olympic sports is because it is also a combination of physics and craftsmanship. I am a science geek and sportsman and have a broad range of interests in science, including physics and engineering.
At the time I took up archery after the 2012 Olympics, I was studying for a degree in physics at the Open University. One of the topics I covered was classical mechanics, which included the physics of the mechanism of the bow and arrow. I also spent some time examining the science of archery. For this post, I have decided to combine my love of science and my love of archery to show that archery is a prime example of how brains and brawn work together in harmony. This should set the stigma aside that geeks like me are not cut out for sports.

First of all, let's start with the mechanics of motion. When you fire a projectile like an arrow, do you expect it to go straight toward the target or up and down in a curved line known as a parabola? Well, for thousands of years, people used to assume the former as the great philosopher Aristotle, tutor to Alexander the Great, told of how projectile motion worked without experimenting with it. He lived in the 4th century BC Macedonia, but he was originally from Greece, where his tutor was Plato. The physicists of Aristotle's day and age didn't experiment with nature very much because they were theorists who used common sense from observation, where logic is impeccable.
Aristotle believed that force was always needed to make an object move until it came to 'a natural state of rest,' as he called it. Arrows are made of naturally heavy materials, so their natural rest state is on the ground. By that logic, when we fire arrows, they will continue to go on in the direction of the target and then land on the ground in a straight direction. Just aim at the target, and once you release the arrow, it will fall to the ground. He later tried to explain how the arrow flies through the air when it doesn't fall directly to the ground upon release. Once again, without experimentation but by observation.



According to Aristotle, the air around the arrow experienced a force from the firing of the bow. The arrow would travel the straightest path that it was set to take by the archer, and in that case, the arrow would always hit the target if aimed in a straight direction. That is not true; if it were, then I would be able to hit the gold ring and score a perfect round, and everyone could be an Olympian.
An Islamic scholar and a French philosopher later trained to expand on Aristotle's science by explaining the force on the arrow was its impetus and fell to the ground once it lost its impetus. This was supposed to explain how an arrow can fall to the ground when shot directly into the sky at an angle and then fall directly to the ground. Later it was realized that this impetus is actually called momentum, best described as the force when the arrow's mass is multiplied by the speed it travels. It wasn't until the 17th century, when scientists like Galileo Galilei finally applied experimentation to all the teachings of the ancients, that the ground rules for physics were established. This meant that from now on, experimentation, practice as well as observation were compulsory for testing scientific theories and technological innovations.
One of Galileo's most significant discoveries about projectile motion would also become Issac Newton's first law of gravity: inertia. That is the reaction an arrow gets when you release the string. The string will bounce back, and the limbs will flex back into their natural shapes, but the arrow can separate away from the string and fly off by the force of the potential power of the limbs and the string. Remember that the bow's power is in the limb's draw weight. The stronger the tension they have when pulled, the heavier their force on the arrow when released back into their standard shape.
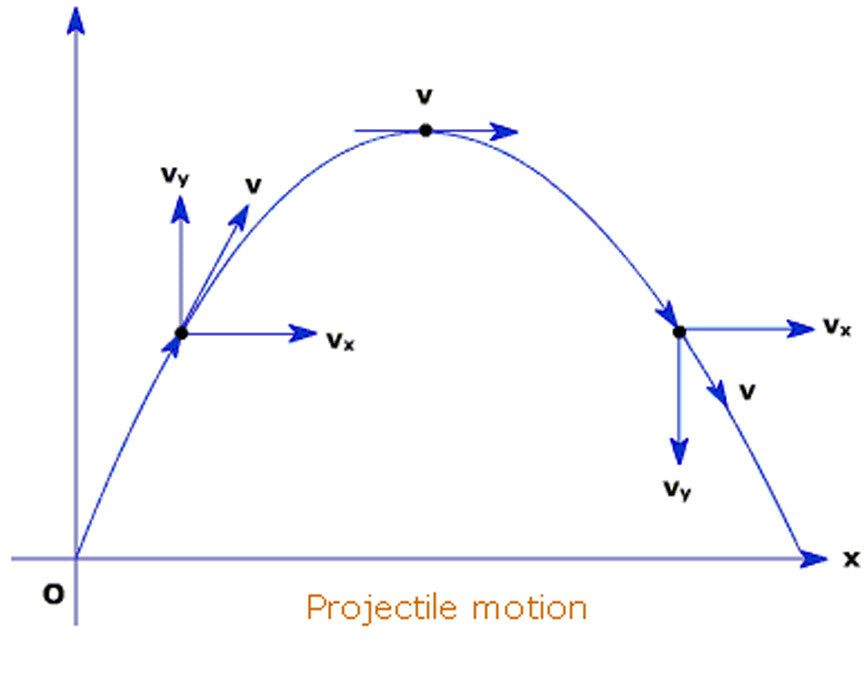
Now Galileo was perhaps most famous for proving that the Earth orbited around the Sun and disproved that the Earth was not at the center of the Universe. Part of this science involved studying the behavior of falling objects and seeing how gravity affects the path they take when they plummet to the ground. He found that all projectiles like arrows don't actually travel in a straight line at all; they travel in a parabola. A parabola is a curved line that starts from one point, goes up, and then comes back down at another point at a distance from the starting point on the same level.
An archer fires the bow and arrow, which travels upward toward an object. Then as soon as it slows down, gravity makes it fall back down again, hitting the target just before it hits the ground altogether. Providing the poundage is suitable for the distance you are shooting at. This is a crucial factor in target archery when you have to direct your bow using the sight and anticipate where it will hit based on how to level the target center with your line of fire. That's why the common bow sight that we have on recurve and compound bows are adjustable for all sizes of the archer, as well as how far the target is and the bow's poundage.
Kinetic Energy and Elastic Potential Energy
Now that we understand how arrows fly the way they do, it might be worth looking into how bows behave. Bows are cleverly crafted pieces of engineering in style and simplicity. Da Vinci had a saying, 'Simplicity is the ultimate sophistication.' A bow's mechanics and physical behavior can be understood as an elegant tool of beauty and precision. A bow is a device that converts slow and steady human force over a distance into stored mechanical potential energy. The stored energy is in the limbs of the bow. This energy is converted into kinetic energy upon release of the bowstring, and a great deal of that kinetic energy is transferred to the arrow shaft. Potential energy is the energy that an object has based on its position and kinetic energy is energy through motion.
When you pull back on your bow, you apply a force to the bow string, which in turn bends the bow as it adds elastic potential energy. Thus a bow is basically a sprint that stores energy to be put into the arrow. The stretched shape of the bow is an example of potential stored energy. Then there's the kinetic energy, which becomes when the string springs back into its normal shape. As the second law of aerodynamics states, energy cannot be created nor destroyed; it just goes somewhere. That energy has now gone into the arrow shaft. When the arrow leaves the bow, it's full of energy, taking its trajectory on a path toward the aim. The only thing that can affect its flight is air resistance and gravity.


Draw Weight and Draw Curve
The bow's full draw weight is often associated with just the bow's limbs. But actually, it's more to do with the length of the bow itself. Draw weight is directly proportional to draw length, as the bow behaves like a spring. Robert Hooke was a scientist who was studying the laws of motion using springs, and he provided some inspiration to Isaac Newton. In 1660 he discovered the law of elasticity which bears his name: Hooke's Law.
Hooke's Law states that the amount of stretch in a spring is proportional to the force pulling on the spring. This can also be applied to bows, where it's known as elastic potential energy. When you pull the bow outwards, the length of the stretch is the same as the force of you pulling it outwards. The force upon the bow is recognized as the draw curve. The draw force curve is slight because of the shape of the bow. Still, when you combine Hooke's Law with other factors, it becomes defined graphically to help understand the relationship between the draw length and the draw weight.


The relationship between the length and the weight of the draw is graphically defined as a straight-line relationship where the energy stored in the bow is calculated using the equation: draw weight multiplied by the draw length divided by the number of limbs (2). For example, my bow has 28 lbs limbs, and I have a draw length of about 28 inches. So 28 lbs x 28 ins/2 = 392 pounds per square inch.
For a recurve bow, the draw weight is defined graphically as a straight line, but a compound bow is defined as a curved line. This is because compound bows work using levers and cams, which decrease the draw weight with the draw length, allowing the bow with the same amount of energy to require less draw force to pull on it and improving bow efficiency. This is why compound bows feel lighter to pull than recurve bows.
This is about as much as I can cover for now. I could go on, but it would be too much for one post, so I have decided to make it in parts. I enjoyed writing this one because it gave me a chance to go over my old textbooks and reading material on physics, trajectory, and engineering principles. This could show that science in sports is not just for the athlete's physical attributes but also work for the performance of the equipment they use. When you understand the laws of physics, you can blend in with the nature of the competition.
 cust@legendarchery.com
cust@legendarchery.com 302 503 5767
302 503 5767 Whitestown, In 47075
Whitestown, In 47075